Komplexe Zahlen
1) Das Symbol "i":
x² = a G = R
a) a > 0
L=
b) a = 0
L=
c) a < 0
L=
T C komplexe Zahlen
x² = -a ; a>0
x² = a (-1)
x1,2= [a]
L=
Definition: [-1] = i
[-1] = i
i² = (
i² = -1
Vorsicht:
ax²+bx+x=0 a,b,c I R; a allg. quadratische Gleichung
x1,2= [-b [b²-4ac]] /[2a] = - [b] /[2a] [b²-4ac]] /[2a]
G = C
a) D = b²-4ac > 0
L=
b) D = 0
L=
c) D < 0
T 4ac-b² > 0
L=
allgemeine komplexe Zahl:
Z = a + b i a,b I R
a = Re (Z) b = Im (Z)
a) b=0 T Z=a+0i .. reelle Zahl
b) a=0 T Z=0+bi .. imaginäre Zahl
Gleichheit von komplexen Zahlen:
Z1 = a+bi
Z2 = c+di
Z1 = Z2 (a=c) (b=d)
2) Rechenregeln für komplexe Zahlen:
Z1 = a + b i Z2 = c + d i
Addition:
Z1 + Z2 = a+bi+c+di = (a+c) + (b+d)i
Subtraktion:
Z1 - Z2 = (a-c) + (b-d)i
Multiplikation:
Z1 * Z2 = (a+bi) (c+di) = ac+adi+bci+bdi² = (ac-bd) + (bc+ad)i
Division:
Z1 : Z2 = [Z1]/[Z2] = [a+bi] /[c+di] = [a+bi] /[c+di] [c-di] /[c-di] = [ac+bci-adi-bdi²] /[c²+d²] =
= [(ac+bd)+(bc-ad)i] /[c²+d²] = [ac+bd] /[c²+d²] + [bc-ad] /[c²+d²] i
c²+d² > 0 , sonst c=0,d=0 T Z2=0
Konjugiert komplexe Zahlen:
Z = a + b i Z- = a - b i
Potenzen von i:
i1 = i
i² = -1
i3 = i² * i = -1 * i = -i
i4 = i² * i² = (-1) * (-1) = 1
Eigenschaften von konjugiert komplexen Zahlen:
Z + Z- = 2a
Z - Z- = 2bi
Z * Z- = a² + b²
(Z-)- = Z
Für komplexe Zahlen gilt auch der Satz von VIETA:
z²+pz+q=0 p,q I C
mit Lösungen z1,z2
a) z1 + z2 = -p
b) z1 * z2 = q
c) z²+pz+q = (z-z1) (z-z2)
3) Veranschaulichung von komplexen Zahlen in der GAUSSschen Zahlenebene:
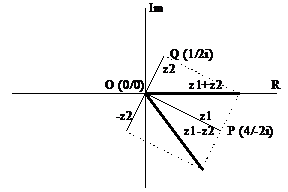
R .. reelle Achse
Im imaginäre Achse
z = a + bi
z1 = 4 - 2i
z1- = 4 + 2i . um R-Achse spiegeln
z2 = 1 + 2i
z1 +z2 = 5
z1 - z2 = z1 + (-z2) = 3 - 4i
Jede komplexe Zahl läßt sich eindeutig als Vektor in der GAUSSschen Zahlenebene darstellen.
| z | = [a²+b²] = r I R . Radius
| z |² = | z² |
4) Darstellungsmöglichkeiten komplexer Zahlen:
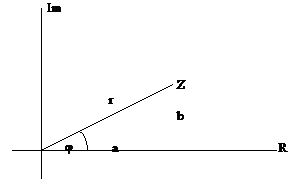
a) z = a + bi
b) z = (a;b)
c) z = (r;j
Polarkoordinaten:
r= [a²+b²]
0<j<
r .. Betrag von z
j . Argument von z
d) tan j = b/a
cos j = a/r
a = r cos j
sin j = b/r
b = r sin j
z = a + bi = r cos j + r sin j i = r (cos j + i sin j
Darstellungsmöglichkeiten komplexer Zahlen:
1) kartesische Darstellung:
a) Zahlenpaar z = (a;b)
b) Binomialform z = a + bi
2) Polarkoordinatendarstellung:
a) Zahlenpaar z = (r; j
b) trigonometrische Darstellung z = r (cos j + i sin j
Multiplikation und Division komplexer Zahlen mit Hilfe von Polarkoordinaten:
z1 = r1 (cos j1 + i sin j
z2 = r2 (cos j2 + i sin j
z1 * z2 = r1 (cos j1 + i sin j1) r2 (cos j2 + i sin j
= r1 * r2 (cos j1 cos j2 - sin j1 sin j2 + cos j1 sin j2 i + sin j1 cos j2 i) =
= r1 * r2 [ (cos j1 cos j2 - sin j1 sin j2) + i (cos j1 sin j2 + cos j2 sin j
= r1 * r2 [cos (j j2) + i sin j j
z1 * z2 = (r1; j1) (r2; j2) = (r1*r2; j j
Beim Multiplizieren von komplexen Zahlen werden die Radien multipliziert und die Winkel addiert.
z1/z2 = [r1 (cos j1 + i sin j /[r2 (cos j2 + i sin j
= [r1 (cos j1 + i sin j1) (cos j2 - i sin j /[r2 (cos j2 + i sin j2) (cos j2 - i sin j
= [r1 (cos j1 cos j2 + i sin j1 cos j2 - i sin j2 cos j1 - i² sin j1 sin j /[r2 (cos² j2 + sin² j
= [r1 [(cos j1 cos j2 + sin j1 sin j2) + i (sin j1 cos j2 - cos j1 sin j /[r2 (cos² j2 + sin² j
= r1/r2 [cos (j j2) + i sin (j j
z1/z2 = (r1; j1)/(r2; j2) = (r1/r2; j j
Beim Dividieren von komplexen Zahlen werden die Radien dividiert und die Winkel subtrahiert.
Haupt | Fügen Sie Referat | Kontakt | Impressum | Nutzungsbedingungen
